Class 5
Fraction - Addition & Subtraction
Division of fraction
When you divide a fraction by another number (whole number or fraction), you convert the division into multiplication. To do this, you flip the second number (take its reciprocal) and change the division sign to multiplication.
Steps to Divide Fractions
Write the problem: Start with the division problem.
Reciprocal: Flip the second fraction (swap numerator and denominator).
Change to multiplication: Replace the division sign (÷) with a multiplication sign (×).
Multiply: Multiply the numerators and multiply the denominators.
Simplify: Simplify the result if possible.
Examples
1. Dividing a Fraction by a Whole Number
Example: 1/2÷3
Write the problem: 1/2÷3
Turn the whole number into a fraction: 3=3/1
Flip the second fraction: 3/1 becomes 1/3
Change to multiplication:
1/2÷3/1 becomes 1/2×1/3
Multiply:
Numerator: 1×1=11 \times 1 = 1
Denominator: 2×3 = 6
Result: 1/6
Answer: 1/2÷3=1/6
2. Dividing a Fraction by Another Fraction
Example: 3/4÷1/2
Write the problem: 3/4÷1/2
Flip the second fraction: 1/2 becomes 2/1
Change to multiplication:
3/4÷1/2 becomes 3/4×2/1
Multiply:
Numerator: 3×2=6
Denominator: 4×1=4
Result: 6/4
Simplify: 6/4=3/2 or 1 1/2 (mixed fraction).
Answer: 3/4÷1/2=3/2 or 1 1/2
Important Points to Remember
Flip the second fraction (reciprocal) when dividing.
Change division to multiplication.
Comparing Unlike Fractions (Fractions with Different Denominators)
Steps to Compare Unlike Fractions
Understand the fractions: Write down the fractions you want to compare.
Find the LCM (Least Common Multiple) of the denominators. This helps to make the denominators the same.
Convert the fractions: Rewrite each fraction with the same denominator by multiplying the numerator and denominator by appropriate numbers.
Compare the numerators: Once the denominators are the same, compare the numerators.
The fraction with the larger numerator is the bigger fraction.
Simplify the answer if required.
Examples
1. Compare 2/3 and 3/4
Write down the fractions: 2/3 and 3/4.
Here, the denominators are 3 and 4 (unlike denominators).
Find the LCM of 3 and 4:
Multiples of 3: 3, 6, 9, 12…
Multiples of 4: 4, 8, 12…
LCM = 12
Convert both fractions to have the same denominator (12):
For 2/3: Multiply numerator and denominator by 4 → 2×4/3×4=8/12
For 3/4: Multiply numerator and denominator by 3 → 3×3/4×3=9/12
Compare the numerators:
8/12 and 9/12
Since 9 > 8, 9/12 is greater.
Simplify and write the answer:
3/4>2/3
2. Compare 1/2 and 3/5
Write down the fractions: 1/2 and 3/5.
Find the LCM of 2 and 5:
Multiples of 2: 2, 4, 6, 8, 10…
Multiples of 5: 5, 10…
LCM = 10
Convert both fractions to have the same denominator (10):
For 1/2: Multiply numerator and denominator by 5 → 1×5/2×5=5/10
For 3/5: Multiply numerator and denominator by 2 → 3×2/5×2=6/10
Compare the numerators:
5/10 and 6/10
Since 6 > 5, 6/10 is greater.
Simplify and write the answer:
3/5 > 1/2
Odering like fraction with equal numerator
When ordering fractions with equal numerators, you are arranging them from smallest to largest (ascending) or largest to smallest (descending) based on their denominators. Here’s an easy explanation for Class 5 students:
Understanding the Rule:
If two or more fractions have the same numerator (top number), the size of the fraction depends on the denominator (bottom number). The larger the denominator, the smaller the fraction.
Example:
Let’s compare these fractions:
2/3,2/5,2/7,2/9
Here, the numerator (2) is the same for all fractions.
To order them:
Look at the denominators: 3, 5, 7, 9.
Larger denominators mean smaller fractions.
2/9
2/3
Ordered from Smallest to Largest:
2/9,2/7,2/5,2/3
Key Tip to Remember:
If numerators are equal:
Compare the denominators.
Smaller denominator → Larger fraction.
Odering unlike fraction with unequal numerator
Steps to Order Unlike Fractions with Unequal Numerators
Understand the Problem:
Unlike fractions have different denominators (e.g., 23,34,56\frac{2}{3}, \frac{3}{4}, \frac{5}{6}).
Here, both the numerators and denominators are not the same.
Find the LCM of the Denominators:
Find the Least Common Multiple (LCM) of the denominators to make the fractions have the same denominator.
Example: For 23,34,56\frac{2}{3}, \frac{3}{4}, \frac{5}{6}, the denominators are 3, 4, and 6. The LCM is 12.
Convert Fractions to Equivalent Fractions:
Multiply the numerator and denominator of each fraction so that the denominators become the same (the LCM).
Example:
For 23\frac{2}{3}, multiply by 4: 2×43×4=812\frac{2 \times 4}{3 \times 4} = \frac{8}{12}.
For 34\frac{3}{4}, multiply by 3: 3×34×3=912\frac{3 \times 3}{4 \times 3} = \frac{9}{12}.
For 56\frac{5}{6}, multiply by 2: 5×26×2=1012\frac{5 \times 2}{6 \times 2} = \frac{10}{12}.
Compare the Numerators:
Once the denominators are the same, compare the numerators to order the fractions.
812,912,1012\frac{8}{12}, \frac{9}{12}, \frac{10}{12}:
Ascending Order: 812<912<1012\frac{8}{12} < \frac{9}{12} < \frac{10}{12}.
Original fractions: 23<34<56\frac{2}{3} < \frac{3}{4} < \frac{5}{6}.
Write the Final Answer:
The fractions are now ordered. If asked for descending order, reverse the result.
Addition and subtraction of number
In fractions, addition and subtraction can be done in two main cases:
Fractions with the same denominators (like fractions)
Fractions with different denominators (unlike fractions)
Let’s understand both step by step in a very simple way:
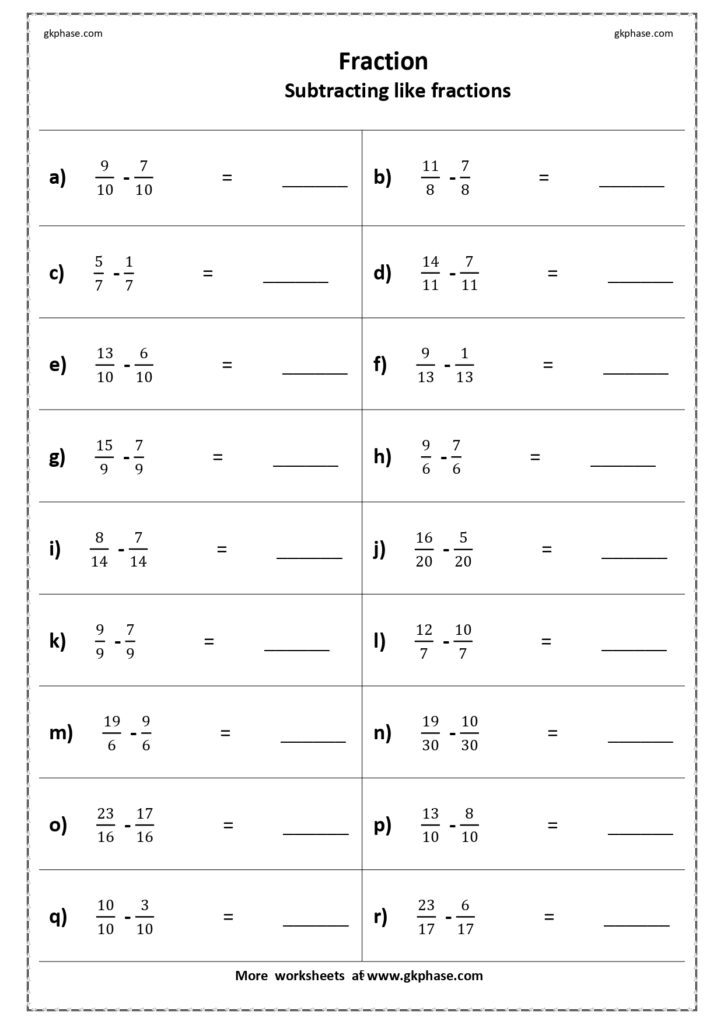
1. Addition and Subtraction of Like Fractions (Same Denominators):
When fractions have the same denominators, you only need to add or subtract the numerators. The denominator remains the same.
Steps:
Add or subtract the numerators.
Keep the denominator the same.
Simplify the fraction if needed.
Example 1: Addition of Like Fractions
3/7+2/7
Add the numerators: 3+2=5
Keep the denominator the same: 7
Result: 5/7.
Example 2: Subtraction of Like Fractions
5/8−3/8
Subtract the numerators: 5−3=2.
Keep the denominator the same: 8
Result: 2/8.
Simplify the fraction: 2/8=1/4.
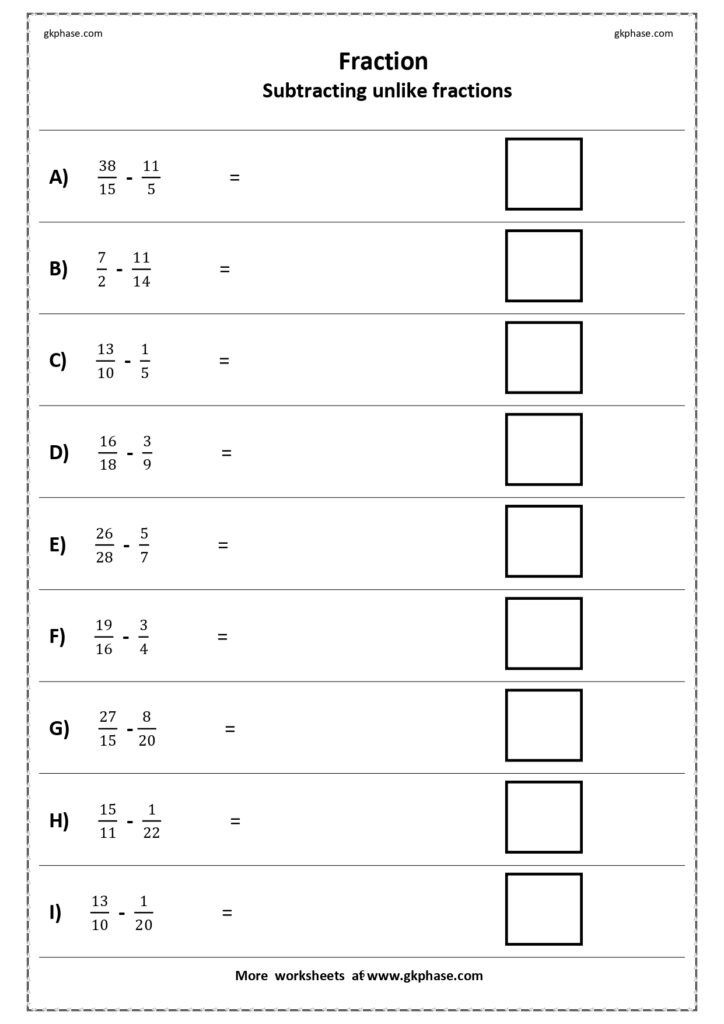
2. Addition and Subtraction of Unlike Fractions (Different Denominators):
When fractions have different denominators, you need to make the denominators the same first. This is done by finding the Least Common Multiple (LCM) of the denominators.
Steps:
Find the LCM of the denominators to make them the same.
Convert each fraction to an equivalent fraction with the same denominator.
Add or subtract the numerators.
Keep the denominator unchanged.
Simplify the answer, if possible.
Example 3: Addition of Unlike Fractions
1/3+1/4
Find the LCM of 3 and 4: LCM is 12.
Convert both fractions to have the same denominator:
1/3=1×4/3×4=4/12
1/4=1×3/4×3=3/12
Add the numerators: 4+3=7.
Keep the denominator: 12.
Result: 7/12.
Example 4: Subtraction of Unlike Fractions
5/6−1/4
Find the LCM of 66 and 44: LCM is 12.
Convert both fractions to have the same denominator:
5/6=5×2/6×2=10/12
1/4=1×3/4×3=3/12
Subtract the numerators: 10−3=7.
Keep the denominator: 12.
Result: 7/12
Addition and subtraction of whole number
Understanding Whole Numbers and Fractions
– A whole number is a number like ( 0, 1, 2, 3, 4, … ).
– A fraction represents a part of a whole and is written as a/b, where:
– a = numerator (the top number)
– b = denominator (the bottom number)
Adding Whole Numbers and Fractions
When adding whole numbers and fractions, follow these steps:
Steps to Add
1. Write the whole number and the fraction separately.
2. Combine them as a mixed number.
3. If needed, simplify the fraction.
Example 1: Adding 3 and 2/5
– Whole number = 3
– Fraction = 2/5
Solution: Combine the whole number and fraction.
3 + 2/5 = 3 2/5(This is called a mixed number)
\]
Adding Two Mixed Numbers
To add two mixed numbers (whole numbers + fractions):
1. Add the whole numbers.
2. Add the fractions.
3. If the fraction adds up to an improper fraction (numerator > denominator), convert it to a mixed number.
4. Combine the results.
Example 2: Add 2 1/4 and 3 2/4
– Whole numbers: 2 + 3 = 5
– Fractions: 1/4 + 2/4= 3/4
Solution:
\[
2 1/4 + 3 2/4 = 5 3/4.
Subtracting Whole Numbers and Fractions
When subtracting a fraction from a whole number, follow these steps:
Steps to Subtract
1. Borrow 1 from the whole number (if needed) and convert it into a fraction equal to the denominator of the fraction.
2. Subtract the fractions.
3. Combine the result.
Example 3: Subtract 2/4 from 4
1. Borrow 1 from 4 → It becomes ( 3 + 5/5).
2. Subtract the fractions:
5/5- 2/5 = 3/5.
3. Combine: 3 + 3/5.
Solution:
4 – 2/5 = 3 3/5 .
Subtracting Two Mixed Numbers
To subtract two mixed numbers:
1. Subtract the whole numbers.
2. Subtract the fractions. If the first fraction is smaller than the second, borrow 1 from the whole number.
3. Combine the results.
Example 4: Subtract 4 1/6 from 6 2/6
1. Whole numbers: 6 – 4 = 2 .
2. Fractions: 2/6 – 1/6 = 1/6.
Solution:
6 2/6 – 4 1/6 = 2 1/6.
Key Points to Remember
1. Addition of whole numbers and fractions combines them into a mixed number.
2. Subtraction often involves borrowing 1 from the whole number to handle the fractions.
3. Always simplify the fractions after adding or subtracting.
Simplification of fraction
Simplification of a fraction means reducing the fraction to its lowest terms. A fraction is in its simplest form when the numerator (top number) and denominator (bottom number) have no common factors other than 1.
Steps to Simplify a Fraction
1. Find the Greatest Common Factor (GCF) of the numerator and the denominator.
2. Divide both the numerator and denominator by their GCF.
3. Write the simplified fraction.
Example 1: Simplify 12/16
1. Find the GCF of 12 and 16:
– Factors of 12: 1, 2, 3, 4, 6, 12
– Factors of 16: 1, 2, 4, 8, 16
– Common factors: 1, 2, 4
– GCF = 4
2. Divide the numerator and denominator by 4:
12/16 = 12 ÷ 4 / 16 ÷ 4 = 3/4.
3. Simplified fraction: 3/4.
Why Simplify Fractions?
– Simplified fractions are easier to understand and compare.
– They make calculations simpler.
Examples of Simplification
Example 2: Simplify 15/25
1. GCF of 15 and 25:
– Factors of 15: \( 1, 3, 5, 15 \)
– Factors of 25: \( 1, 5, 25 \)
– GCF = 5
2. Divide both by 5:
15/25 = 15 ÷ 5 / 25 ÷ 5 = 3/5.
3. Simplified fraction: 3/5.
Example 3: Simplify 18/24
1. GCF of 18 and 24:
– Factors of 18: \( 1, 2, 3, 6, 9, 18 \)
– Factors of 24: \( 1, 2, 3, 4, 6, 8, 12, 24 \)
– GCF = 6
2. Divide both by 6:
18/24 = 18 ÷ 6 / 24 ÷ 6 = 3/4.
3. Simplified fraction: 3/4.
Special Cases
1. If the numerator is 1, the fraction is already in its simplest form.
Example: 1/7.
2. If the numerator and denominator are the same, the simplified fraction is 1.
Example: 8/8 = 1.
3. If the numerator is 0, the fraction is always 0.
Example: 0/5 = 0 .
Word problems
Here are some word problems based on fractions that will help you understand the concept better:
1. Sharing Equal Parts
Question: Sam had 1 pizza. He cut it into 4 equal parts and ate 2 parts.
– What fraction of the pizza did Sam eat?
– What fraction of the pizza is left?
Solution:
– Total parts = 4
– Parts Sam ate = 2 → 2 / 4 (Simplified as 1 / 2)
– Parts left = ( 4 – 2 = 2 ), so 2/4 = 1/2.
Answer: Sam ate 1/ 2of the pizza, and 1/2 is left.
2. Fraction of a Group
Question: Out of 20 students in a class, 1/4 of the students are wearing glasses.
– How many students are wearing glasses?
Solution:
– Total students = 20
– Fraction wearing glasses = 1/4
– Students wearing glasses = 20 x 1/4 = 5.
Answer: 5 students are wearing glasses.
3. Adding Fractions
Question: John ate 2/5 of a cake, and his sister ate 1/5 of the same cake.
– What fraction of the cake did they eat in total?
Solution:
– John’s part = 2/5
– Sister’s part = 1/5
– Total = ( 2/5+ 1/5) = 3/5.
Answer: They ate 3/5 of the cake in total.
4. Subtracting Fractions
Question: A water tank was full. Sarah used 2 / 7 of the water for her plants.
– What fraction of the water is left in the tank?
Solution:
– Total water = ( 1 ) (whole)
– Water used = 2/7
– Water left = ( 1 – 2/7) = 7/7 – 2/7 = 5/7.
Answer: 5/7 of the water is left in the tank.
5. Fraction of a Quantity
Question: A chocolate bar is divided into 8 equal pieces. Emma ate 3/8 of the bar.
– How many pieces did Emma eat?
Solution:
– Total pieces = 8
– Fraction eaten = 3/8
– Pieces Emma ate = 8 x 3/8 = 3.
Answer: Emma ate 3 pieces of chocolate.
6. Comparing Fractions
Question: A garden has two flower beds. One bed has 3 / 6 of flowers blooming, and the other has 2 / 6 of flowers blooming.
– Which flower bed has more flowers blooming?
Solution:
– Compare 3/6 and 2/6. Since ( 3 > 2 ), 3/6 is greater.
Answer: The first flower bed has more flowers blooming.
7. Real-Life Sharing
Question: Ron and his 3 friends shared a large watermelon equally.
– What fraction of the watermelon did each person get?
Solution:
– Total people = 4
– Each person gets 1/4 of the watermelon.
Answer: Each person gets 1/4 of the watermelon.
8. Adding Mixed Numbers
Question: A baker used 2 1 / 4 kg of flour in the morning and 1 3 / 4kg in the afternoon.
– How much flour did the baker use in total?
Solution:
– Add the whole numbers: 2 + 1 = 3 .
– Add the fractions: 1/4 + 3/4 = 4/4 = 1.
– Total = 3 + 1 = 4 .
Answer: The baker used 4 kg of flour in total.
9. Subtracting Mixed Numbers
Question: A jug had 5 1/2 liters of water. 2 1/2 liters were used.
– How much water is left in the jug?
Solution:
– Subtract the whole numbers: 5 – 2 = 3 .
– Subtract the fractions: 1/2 – 1/2 = 0 .
– Total = 3.
Answer: There are 3 liters of water left in the jug.
10. Multiplying Fractions with Whole Numbers
Question: A farmer harvested 1/3 of 21 apples.
– How many apples did the farmer harvest?
Solution:
– Total apples = 21
– Fraction harvested = 1/3
– Apples harvested = 21 x 1/3 = 7 .
Answer: The farmer harvested 7 apples.
Welcome to gkphase
To download free printable activities and worksheet of Fractions (class 5th maths) in PDF form