Factors and Multiples -

Class 5

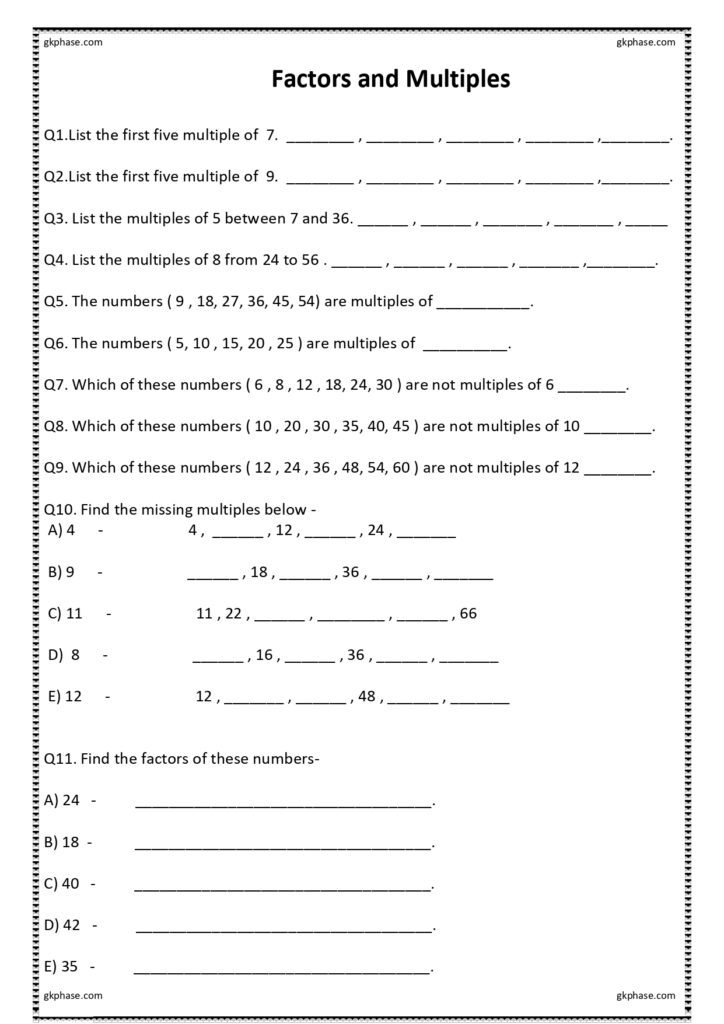
Factors and Multiples

1. Factors

Definition:
A factor is a number that divides another number exactly (without leaving a remainder).

Example:
For the number 12:

Factors of 12 are: 1, 2, 3, 4, 6, 12
Explanation:
- 1 × 12 = 12
- 2 × 6 = 12
- 3 × 4 = 12
- These pairs show that 1, 2, 3, 4, 6, and 12 divide 12 completely.

Properties of Factors:

Every number has at least two factors: 1 and itself.
Factors are always less than or equal to the number.
The smallest factor of any number is 1, and the largest is the number itself.
Factors are whole numbers.

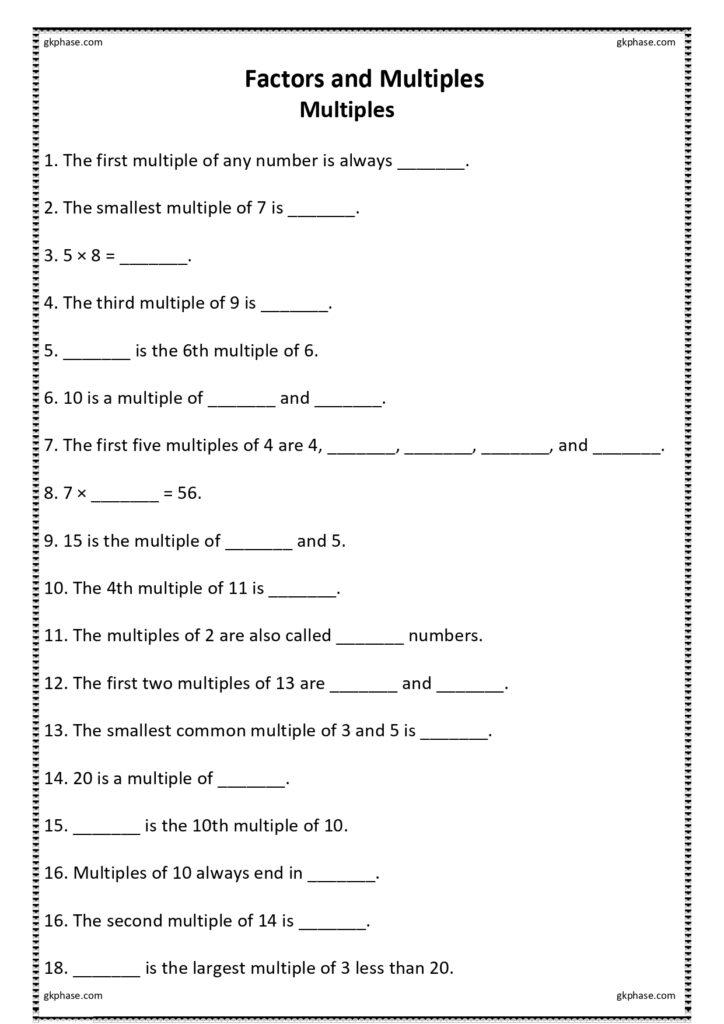
2. Multiples

Definition:
A multiple is the result of multiplying a number by an integer.

Example:
For the number 5:

Multiples of 5 are: 5, 10, 15, 20, 25, 30, … (and so on)
Explanation:
- 5 × 1 = 5
- 5 × 2 = 10
- 5 × 3 = 15
- These are all multiples of 5.

Properties of Multiples:

Every number has infinite multiples.
The smallest multiple of any number is the number itself.
Multiples are greater than or equal to the number.
Zero is a multiple of every number (e.g., 5 × 0 = 0).

Difference Between Factors and Multiples:

Aspect	Factors	Multiples
Definition	Numbers that divide another number exactly.	Numbers obtained by multiplying a number.
Example	Factors of 10: 1, 2, 5, 10	Multiples of 4: 4, 8, 12, 16, 20, …
Number Count	Finite (limited)	Infinite (unlimited)
Size	Smaller than or equal to the number.	Greater than or equal to the number.

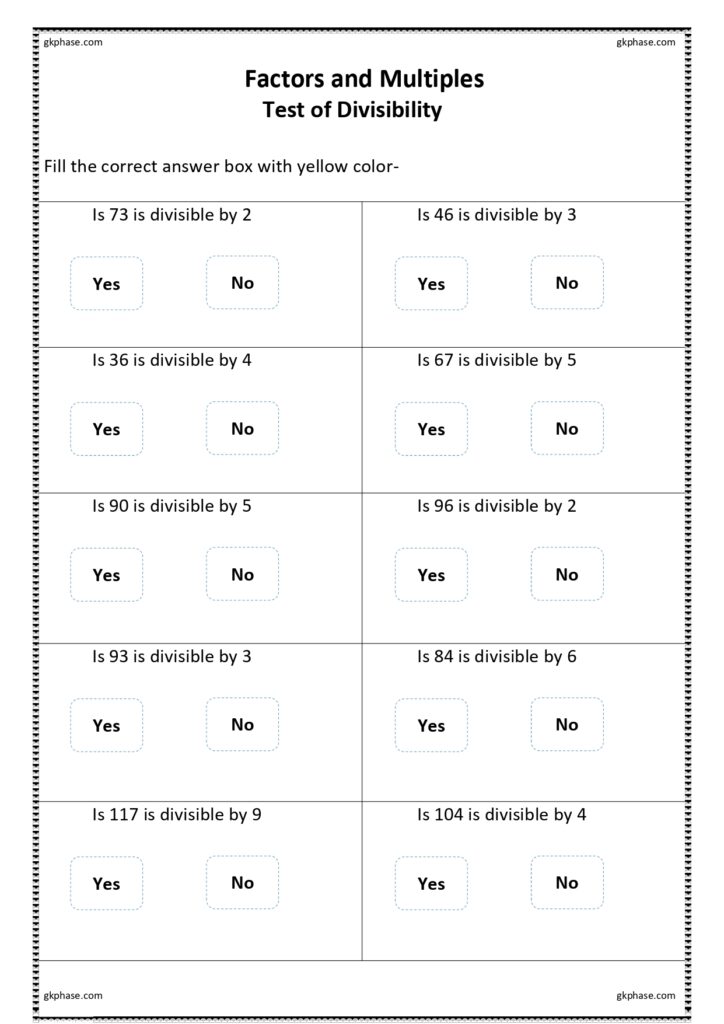
Test of divisibility

Divisibility Rules:

1. Divisibility by 2

Rule: A number is divisible by 2 if its last digit is 0, 2, 4, 6, or 8.
Example:
- 14 → Last digit is 4 → Divisible by 2
- 27 → Last digit is 7 → Not divisible by 2

2. Divisibility by 3

Rule: A number is divisible by 3 if the sum of its digits is divisible by 3.
Example:
- 123 → Sum of digits: 1 + 2 + 3 = 6 → 6 is divisible by 3 → 123 is divisible by 3
- 145 → Sum of digits: 1 + 4 + 5 = 10 → 10 is not divisible by 3 → 145 is not divisible by 3

3. Divisibility by 4

Rule: A number is divisible by 4 if the last two digits form a number divisible by 4.
Example:
- 316 → Last two digits: 16 → 16 ÷ 4 = 4 → Divisible by 4
- 725 → Last two digits: 25 → 25 ÷ 4 = 6 remainder 1 → Not divisible by 4

4. Divisibility by 5

Rule: A number is divisible by 5 if its last digit is 0 or 5.
Example:
- 45 → Last digit is 5 → Divisible by 5
- 102 → Last digit is 2 → Not divisible by 5

5. Divisibility by 6

Rule: A number is divisible by 6 if it is divisible by both 2 and 3.
Example:
- 72 → Divisible by 2 (ends in 2) and by 3 (7 + 2 = 9) → Divisible by 6
- 25 → Not divisible by 2 → Not divisible by 6

6. Divisibility by 8

Rule: A number is divisible by 8 if the last three digits form a number divisible by 8.
Example:
- 1,000 → Last three digits: 000 → 0 is divisible by 8 → Divisible by 8
- 234 → Last three digits: 234 → 234 ÷ 8 = 29 remainder 2 → Not divisible by 8

7. Divisibility by 9

Rule: A number is divisible by 9 if the sum of its digits is divisible by 9.
Example:
- 729 → Sum of digits: 7 + 2 + 9 = 18 → 18 is divisible by 9 → 729 is divisible by 9
- 123 → Sum of digits: 1 + 2 + 3 = 6 → 6 is not divisible by 9 → Not divisible by 9

8. Divisibility by 10

Rule: A number is divisible by 10 if its last digit is 0.
Example:
- 150 → Last digit is 0 → Divisible by 10
- 123 → Last digit is 3 → Not divisible by 10

9. Divisibility by 11

Rule: A number is divisible by 11 if the difference between the sum of digits in odd positions and the sum of digits in even positions is divisible by 11 (including 0).
Example:
- 121 → (1 + 1) – 2 = 0 → 0 is divisible by 11 → 121 is divisible by 11
- 234 → (2 + 4) – 3 = 3 → Not divisible by 11

H.C.F (Highest common factor)

The Highest Common Factor (HCF) of two or more numbers is the largest number that divides each of them exactly without leaving a remainder. It’s also called the Greatest Common Divisor (GCD).